Question

What is the vertex form equation of a parabola with vertex (2, -5) that goes through point (-2, 7)

Answer 1

`y=(3)/(4)(x-2)^2-5`

Explanation:

`\boxed{\begin{minipage}{5.6 cm}\underline{Vertex form of a quadratic equation}\\\\$y=a(x-h)^2+k$\\\\where:\\ \phantom{ww}$\bullet$ $(h,k)$ is the vertex. \\ \phantom{ww}$\bullet$ $a$ is some constant.\\\end{minipage}}`

Given:

• Vertex = (2, -5)
• Point = (-2, 7)

Substitute the given vertex and point into the formula and solve for a:

`\implies 7=a(-2-2)^2+(-5)`

`\implies 7=a(-4)^2-5`

`\implies 7=16a-5`

`\implies 12=16a`

`\implies a=(12)/(16)`

`\implies a=(3)/(4)`

Substitute the vertex and the found value of a into the formula to create an equation in vertex form for the given parameters:

`y=(3)/(4)(x-2)^2-5`

Answer 2

y =
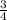`(3)/(4)` (x - 2)² - 5

Explanation:

the equation of a parabola in vertex form is

y = a(x - h)² + k

where (h, k ) are the coordinates of the vertex and a is a multiplier

here (h, k ) = (2, - 5 ) , then

y = a(x - 2)² - 5

to find a substitute (- 2, 7 ) into the equation

7 = a(- 2 - 2)² - 5 ( add 5 to both sides )

12 = a(- 4)² = 16a ( divide both sides by 16 )

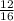`(12)/(16)` = a , that is a =
`(3)/(4)`

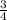
y =
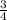`(3)/(4)` (x - 2)² - 5 ← equation in vertex form