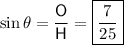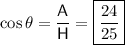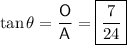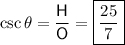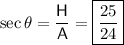Answer:
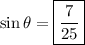
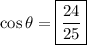


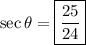
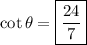
Explanation:
Trigonometric ratios (right triangle)

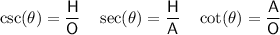
where:
- θ is the angle.
- O is the side opposite the angle.
- A is the side adjacent the angle.
- H is the hypotenuse (the side opposite the right angle).
Given:
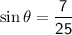
Therefore:
- The side opposite the angle is 7.
- The hypotenuse of the right triangle is 25.
Find the measure of the other leg of the right triangle by using Pythagoras Theorem:
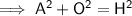
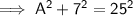

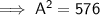
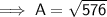

Therefore:
The exact values of the trigonometric functions are: