Answer:
Approximately
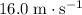 .
.
Step-by-step explanation:
When an object of mass
 travels at a velocity of
travels at a velocity of
 , the momentum
, the momentum
 of that object will be
of that object will be
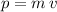 .
.
In this example, the momentum of the car before the collision will be:
 .
.
Since the truck was initially not moving, the initial momentum of the truck will be
 .
.
Momentum is conserved in collisions. In other words, the sum of the momentum of the truck and the car will be the same right before and after the collision.
The sum of the momentum of the truck and the car was approximately
 right before the collision. By the conservation of momentum, the sum of the momentum of the two vehicles right after the collision will also be
right before the collision. By the conservation of momentum, the sum of the momentum of the two vehicles right after the collision will also be
 .
.
The velocity of the two vehicles right after the collision will be the same since the vehicles are stuck together. Let
 denote this velocity.
denote this velocity.
The sum of the mass of the two vehicles is
 . Divide the total momentum of the two vehicles by their total mass to find the velocity:
. Divide the total momentum of the two vehicles by their total mass to find the velocity:
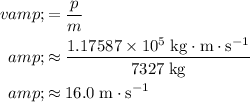 .
.