Answer:
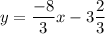
Explanation:
Slope-intercept form is y = mx + b. This is where m is the slope and b is the y-intercept.
We will be writing a point-slope equation, then simplifying it into a slope-intercept form equation. First, we need to find the slope. We find that we have a slope of
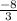 .
.
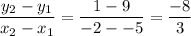
Next, we will write the point-slope equation.
y -
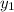 = m(x -
= m(x -
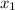 )
)
y - 9 =
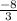 (x - -5)
(x - -5)
y - 9 =
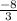 (x + 5)
(x + 5)
y - 9 =
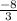 x - 13
x - 13
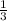
y =
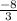 x - 3
x - 3
