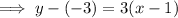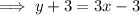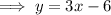Answer:

Explanation:
Given equation of a line:
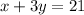
Rearrange the given equation to make y the subject:

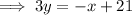
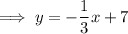
If two lines are perpendicular to each other, their slopes are negative reciprocals.
Therefore, the slope of the perpendicular line is 3.

Substitute the found slope and the given point (1, -3) into the point-slope formula: