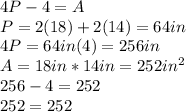Question

The length of a rectangle is 4 inches more than its width. The area of the rectangle is equal to 4 inches less than 4 times the perimeter. Find l and w

Answer 1

Explanation:

`l=w+4`

`A=l*w\\l=w+4\\A=(w+4)w\\A=w^2+4w`

`P=2l+2w\\A=4p-4\\A=4(2l+2w)-4\\A=8l+8w-4\\l=w+4\\A=8(w+4)+8w-4\\A=8w+32+8w-4\\A=16w+28`

`16w+28=A=w^2+4w\\16w+28=w^2+4w\\w^2-12w-28=0\\w^2-14w+2w-28=0\\(w^2-14w)+(2w-28)=0\\w(w-14)+2(w-14)=0\\w+2=0=w-14\\w=-2and14`

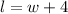
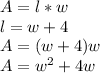
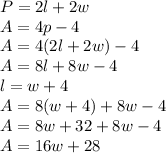
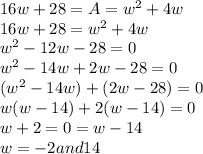
Since w represents a measurement of a physical object in inches, it cannot be negative. So,
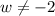`w\\eq-2`. The width of the rectangle is 14 inches.

The length is given as 4 inches more than the width, so the length is 18 inches.

This satisfies all the requirements of the question:

`4P-4=A\\P=2(18)+2(14)=64in\\4P=64in(4)=256in\\A=18in*14in=252in^2\\256-4=252\\252=252`