Answer:
x^2(3x-2) cubic inches OR in^3
OR
3x { 3 [ 3x ( x - 2 ) + 4 ] } - 8 cubic inches OR in^3
I AM UNAWARE IF YOU ASKED THAT ONE SIDE IS (3X-2) OR ALL. I WILL ANSWER BOTH PARTS
-
NOTE: '^' MEANS TO THE POWER OF..
-
Volume = v, abc = 3 sides of cube (height, width, length)
Using the formula for volume in a cube,
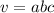
We can solve this.
If one side is (3x-2)in,
- (3x-2)(x)(x) = v.... x are the other two sides
- x^2(3x-2) = v
x^2(3x-2) cubic inches OR in^3
If all sides are (3x-2)in,
Use the formula,
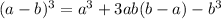
We can solve this.
- (3x-2)(3x-2)(3x-2) = v
- (3x-2)^3 = v.... 3x = a and -2 = b
- (3x)^3 + [(3)(3x)(2)][2-3x] - (2)^3 = v
- 27x^3 + 18x(2-3x) -8 = v
- (27x^3 + 36x - 54x^2) - 8 = v.. Terms inside brackets - take 3x as common and leave out 8
- 3x(9x^2 -18x +12) = v... Take 3 as common again in the brackets
- 3x [ 3 ([3x^2 -6x] + 4) -8 = v....Take 3x common in the terms in square brackets
- 3x [ 3 [ 3x (x-2) + 4 ]] - 8 = v
- 3x { 3 [ 3x ( x - 2 ) + 4 ] } - 8 = v
3x { 3 [ 3x ( x - 2 ) + 4 ] } - 8 cubic inches OR in^3
___
If you have any questions regarding formulas or anything, comment and I will get back to you asap.
___