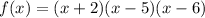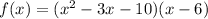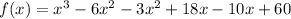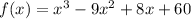Question

Find a polynomial function of degree 3 with the given numbers as zeros. Assume that the leading coefficient is 1.

-2,5,6

Answer 1

`f(x)=x^3-9x^2+8x+60`

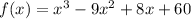
Explanation:

The zeros of a function are the x-values when f(x) = 0.

Factor Theorem

If f(x) is a polynomial, and f(a) = 0, then (x – a) is a factor of f(x).

If the zeros of the polynomial are -2, 5 and 6 then (x + 2), (x - 5) and (x - 6) are factors of the polynomial.

Therefore, the polynomial in factored form is:

`f(x)=a(x+2)(x-5)(x-6)`

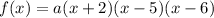
where a is the leading coefficient.

Given a = 1:

`f(x)=(x+2)(x-5)(x-6)`

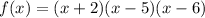
Expand the parentheses to express the polynomial in standard form:

`f(x)=(x+2)(x-5)(x-6)`

`f(x)=(x^2-3x-10)(x-6)`

`f(x)=x^3-6x^2-3x^2+18x-10x+60`

`f(x)=x^3-9x^2+8x+60`