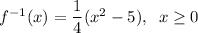Answer:
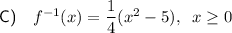
Explanation:
Given function:

The domain of the given function is restricted to x ≥ -⁵/₄.
Therefore, the range of the given function is restricted to f(x) ≥ 0.
The inverse of a function is its reflection in the line y = x.
To find the inverse of a function, swap x and y:
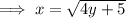
Rearrange the equation to make y the subject:
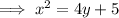
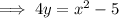
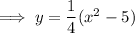
Replace y with f⁻¹(x):
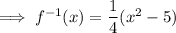
The domain of the inverse of a function is the range of the original function.
Therefore, the domain of the inverse function is x ≥ 0.
Therefore, the inverse of the given function is: