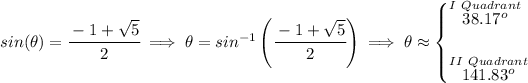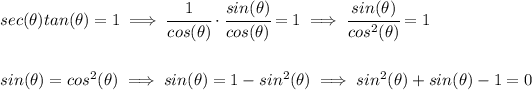
well, it doesn't quite factor into neat integers, so let's plug it in the quadratic formula
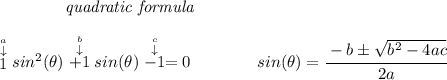
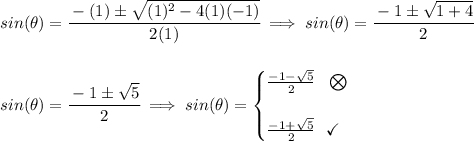
now, why is the 1st value not valid? well, the 1st value gives us a value that's over -1, about -1.62, and the sine function is always -1 ⩽ sine ⩽ 1, so we can't use that, so let's use the 2nd value which is within range