Question

Find an equation for the perpendicular bisector of the line segment whose endpoints

are
(8,1) and (-4, 9).

Answer 1

well, we know the line is a bisector to that segment with those points, hmmmm let's check the midpoint of that segment anyway, since a bisector will hit it at the midpoint

`~~~~~~~~~~~~\textit{middle point of 2 points } \\\\ (\stackrel{x_1}{8}~,~\stackrel{y_1}{1})\qquad (\stackrel{x_2}{-4}~,~\stackrel{y_2}{9}) \qquad \left(\cfrac{ x_2 + x_1}{2}~~~ ,~~~ \cfrac{ y_2 + y_1}{2} \right) \\\\\\ \left(\cfrac{ -4 +8}{2}~~~ ,~~~ \cfrac{ 9 +1}{2} \right) \implies \left(\cfrac{ 4 }{2}~~~ ,~~~ \cfrac{ 10 }{2} \right)\implies (2~~,~~5)`

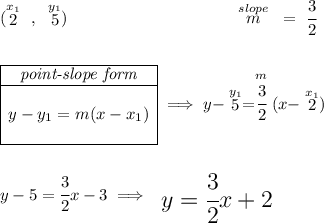
so it passes through (2 , 5), hmmm now, keeping in mind that perpendicular lines have negative reciprocal slopes, let's check for the slope of the segment above

`(\stackrel{x_1}{8}~,~\stackrel{y_1}{1})\qquad (\stackrel{x_2}{-4}~,~\stackrel{y_2}{9}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{rise} {\stackrel{y_2}{9}-\stackrel{y1}{1}}}{\underset{run} {\underset{x_2}{-4}-\underset{x_1}{8}}} \implies \cfrac{ 8 }{ -12 } \implies - \cfrac{2 }{ 3 } \\\\[-0.35em] ~\dotfill`

`\stackrel{~\hspace{5em}\textit{perpendicular lines have \underline{negative reciprocal} slopes}~\hspace{5em}} {\stackrel{slope}{\cfrac{-2}{3}} ~\hfill \stackrel{reciprocal}{\cfrac{3}{-2}} ~\hfill \stackrel{negative~reciprocal}{-\cfrac{3}{-2}\implies \cfrac{3}{2}}}`

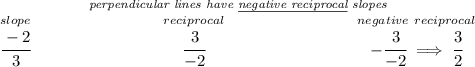
so we're really looking for the equation of a line whose slope is 3/2 and that it passes through (2 , 5)

`(\stackrel{x_1}{2}~,~\stackrel{y_1}{5})\hspace{10em} \stackrel{slope}{m} ~=~ \cfrac{3}{2} \\\\\\ \begin{array} \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{5}=\stackrel{m}{ \cfrac{3}{2}}(x-\stackrel{x_1}{2}) \\\\\\ y-5=\cfrac{3}{2}x-3\implies {\Large \begin{array}{llll} y=\cfrac{3}{2}x+2 \end{array}}`