The maximum value of quadratic function
is at vertex of its parabola.
The maximum value of f(x) = ax² + bx + c is k at x=h
where:
 and k = f(h)
and k = f(h)
We have t instead of x so it's quadratic function named h with the variable t.
So, the function gets the maximum of k at t=h₁
h(t) = -16x² + 70x + 7 ⇒ a = -16, b = 40
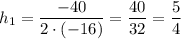
It will take 1.25 s for the rocket to reach its maximum height.
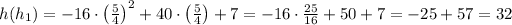
The maximum height is 32 feet.