Question

Help I need it now!!
T14= 46, and t20= 100, find t3, t7, and tn

Answer 1

`t_3 = -53\\\\\\t_7 = -17\\t_n= -80 +9n`

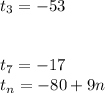
Explanation:

Arithmetic sequence:

`\sf \boxed{t_n=a+(n-1)d}`

Here a is the first term and d is the common difference.

`t_(14)= 6` ⇒
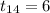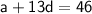`\sf a + 13 d = 46` ------------(I)

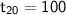`\sf t_(20) = 100` ⇒ a + 19d = 100 ---------(II)

Subtract equation (I) from (II)

(I1) a + 19d = 100

(II) a + 13d = 46

- - -

6d = 54

d = 54 ÷ 6

`\sf \boxed{d = 9}`

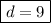
Substitute d = 9 in equation(I) and find 'a',

a + 13*9= 46

a + 117 = 46

a = 46 - 117

a = -71

`\sf t_3 = -71 + 2*9`

= -71 + 18

= -53

`\sf t_7 = -71 + 6*9`

= -71 + 54

= -17

`\sf t_n= -71 + (n-1)*9`

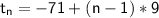
= -71 + 9*n - 1 *9

= -71 + 9n - 9

= -71 - 9 + 9n

= - 80 + 9n