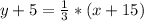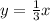Answer:
Option A
The equation of the perpendicular line in slope-intercept-form is
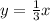
Explanation:
Use the slope-intercept form to find the slope.
The slope-intercept form is y = m x + b , where m is the slope and b is the y-intercept.
Using the slope-intercept form, the slope is − 3 .
The equation of a perpendicular line must have a slope that is the negative reciprocal of the original slope.
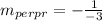
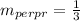
Find the equation of the perpendicular line using the point-slope formula.
Use the slope
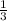 and a given point ( − 15 , − 5 ) to substitute for
and a given point ( − 15 , − 5 ) to substitute for
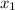 and
and
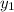 in the point-slope form
in the point-slope form
 .
.
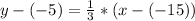
Simplify the equation and keep it in point-slope form.
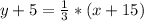
Write in y = m x + b form.
Solve for y .