Question

Please help meee :((

The system of conics has two solutions.

(x−4)2+(y+1)2=9
(x−4)29+(y+1)281=1

What are the solutions to this system of conics?

Answer 1

`\left(\; \boxed{1}\:,\boxed{-1}\;\right)\; \textsf{and}\;\left(\; \boxed{7}\:,\boxed{-1}\;\right)`

Explanation:

Given system of conics:

`\begin{cases}(x-4)^2+(y+1)^2=9\\\\((x-4)^2)/(9)+((y+1)^2)/(81)=1\end{aligned}`

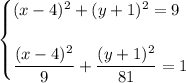
Multiply the second equation by 81:

`\implies (81(x-4)^2)/(9)+(81(y+1)^2)/(81)=81`

`\implies 9(x-4)^2+(y+1)^2=81`

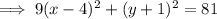
Rearrange to make (y + 1)² the subject:

`\implies (y+1)^2=81-9(x-4)^2`

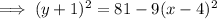
Substitute into the first equation and simplify:

`\implies (x-4)^2+81-9(x-4)^2=9`

`\implies -8(x-4)^2=-72`

`\implies (x-4)^2=9`

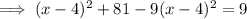
Solve for x:

`\implies √( (x-4)^2)=√(9)`

`\implies x-4=\pm3`

`\implies x=4\pm3`

`\implies x=1, 7`

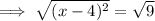
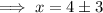
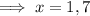
Substitute the found values of x into the first equation and solve for y:

`\begin{aligned}x=1 \implies (1-4)^2+(y+1)^2&=9\\(-3)^2+(y+1)^2&=9\\9+(y+1)^2&=9\\(y+1)^2&=0\\y+1&=0\\y&=-1\end{aligned}`

`\begin{aligned}x=7 \implies (7-4)^2+(y+1)^2&=9\\(3)^2+(y+1)^2&=9\\9+(y+1)^2&=9\\(y+1)^2&=0\\y+1&=0\\y&=-1\end{aligned}`

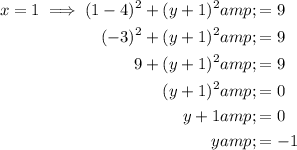
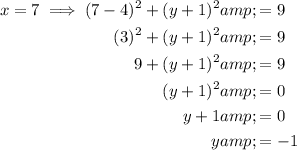
Therefore, the solution to the given system of conics is:

• (1, -1) and (7, -1)

Answer 2

• (1, -1) and (7, -1)

===============

Given system

• (x − 4)² + (y + 1)² = 9
• (x − 4)² / 9 + (y + 1)² / 81 = 1

Solve it by elimination

Multiply the second equation by 9 and subtract from the first one:

• (y + 1)² - (y + 1)² / 9 = 0
• (y + 1)²/8 = 0
• (y + 1)² = 0
• y + 1 = 0
• y = - 1

Substitute y and solve for x

• (x − 4)² + (-1 + 1)² = 9
• (x - 4)² = 9
• x -4 = ± 3
• x = 4 ± 3
• x = 1 and x = 7