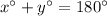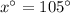2 Answers

Industrial · Answer 1 · 2023-04-25T04:49:57+0000

Answer:

==========================

x is the exterior angle of the triangle.

Exterior angle is the sum of remote interior angles:

Moody · Answer 2 · 2023-04-29T22:02:06+0000

Answer:

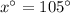
$x\textdegree = 105\textdegree$

Explanation:

To find x, we must first find its corresponding interior angle.

We know that the measures of the interior angles of a triangle add to 180°.

From this, we can construct an equation for x's corresponding interior angle, which I will label y.

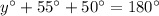
$y \textdegree + 55\textdegree + 50\textdegree = 180\textdegree$

Then, we can solve for y by subtracting 55° and 50° from both sides.

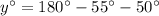
$y\textdegree = 180\textdegree - 55\textdegree - 50\textdegree$

$y\textdegree = 125\textdegree - 50\textdegree$

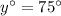
$y\textdegree = 75\textdegree$

Now, we can solve for x, as any interior angle of a triangle and its corresponding exterior angle are supplementary; their measures add to 180°.

$x\textdegree + y\textdegree = 180\textdegree$

$x\textdegree + 75\textdegree = 180\textdegree$

$x\textdegree = 105\textdegree$

Please log in or register to add a comment.