Question

Given: x-1;x+1;x...as the first 3 terms of a geometric sequence

1.1 Determine the value (s)of x
1.2 Hence,or otherwise,find the common ratio of the sequence
1.3why is the sequence convergent​

Answer 1

see explanation

Explanation:

(1)

The common ratio r of a geometric sequence is

r =
`(a_(2) )/(x_(1) )` =
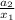`(a_(3) )/(a_(2) )` , so

`(x+1)/(x-1)` =
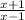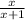`(x)/(x+1)` ( cross- multiply )

(x + 1)² = x(x - 1) ← expand both sides

x² + 2x + 1 = x² - x ( subtract x² - x from both sides )

3x + 1 = 0 ( subtract 1 from both sides )

3x = - 1 ( divide both sides by 3 )

x = -
`(1)/(3)`

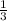
(2)

r =
`(x)/(x+1)` =
`(-(1)/(3) )/(-(1)/(3)+1 )` =
`(-(1)/(3) )/((2)/(3) )` = -
`(1)/(2)`

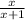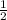
(3)

If - 1 < r < 1 then the sequence will converge

r = -
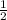`(1)/(2)` meets this criteria , thus the sequence is convergent