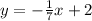Question

Write the equation of the line parallel to x+7y=21 that passes through the point (–14, 4).

Answer 1

⇒Parallel lines have equal gradients meaning the gradient of x+7y=21 is equal to the gradient pf the line that passes through the point (-14,4)

⇒Note to get the gradient of the line x+7y=21 let us first write it in the form y=mx+c as it is not in the correct order of the general equation of a straight line.

`x+7y=21\\7y=21-x\\(7y)/(7) =(21)/(7) -(x)/(7) \\y=3-(x)/(7)`

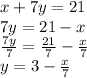
⇒The gradient of the line given as indicated above as we know that is is m which is the coefficient of x is
`-(1)/(7)`

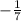
⇒This simply means that the gradient of the new equation is
`-(1)/(7)`

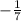
In the general equation y=mx+c let us plug in the known values of the variable and solve for the value of c

We are also given the point (x,y) ⇒ (-14,4)

`4=-(1)/(7) (-14)+c\\4=2+c\\c=4-2\\c=2`

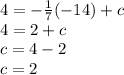
The answer of the line parallel to x+7y=21

`y=-(1)/(7) x+2`