Question

If f(x) = -8m + 3 and g(x) = -8m^2 + m - 5, what is (f times g)x?

A.) 64m^3 - 16m^2 + 43m - 15
B.) 64m^3 +43m - 15
C.) 64m^3 - 32m^2 + 43m - 15
D.) -16m^2 + 43m - 15

Answer 1

Answer: Choice C

`64m^3-32m^2+43m-15`

==================================================

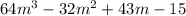
Step-by-step explanation:

Multiply out the expressions using the distribution rule as shown below.

`(-8m+3)(-8m^2+m-5)\\\\n(-8m^2+m-5) \ \ \text{ ... see note1}\\\\-8m^2n+mn-5n\\\\-8m^2( n )+m( n )-5( n )\\\\-8m^2( -8m+3 )+m( -8m+3 )-5( -8m+3 ) \ \ \text{ ... see note2}\\\\( 64m^3-24m^2 )+( -8m^2+3m )+( 40m-15 )\\\\64m^3+(-24m^2-8m^2)+(3m+40m)-15 \\\\64m^3-32m^2+43m-15 \\\\`

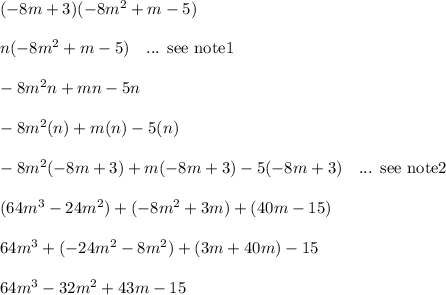
I used the tool WolframAlpha to confirm the answer is correct. GeoGebra is another free tool that offers similar capabilities.

Footnotes:

• Note1: I let n = -8m+3, and replaced -8m+3 with n. That way the distribution on the next step could happen.
• Note2: I plugged in n = -8m+3. After which distribution happens 3 more times.