Answer:
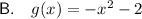
Explanation:
Transformations
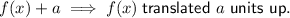


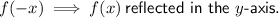
From inspection of the given graph, we can see that g(x) is function f(x) reflected in the x-axis and translated 2 units down.
Given function f(x):

Reflected in the x-axis:
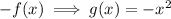
Translated 2 units down:

Therefore:

Note:
If the leading coefficient of a quadratic function is positive, the parabola opens upwards.
If the leading coefficient of a quadratic function is negative, the parabola opens downwards.
Therefore, as g(x) opens downwards, the term in x² will be negative.