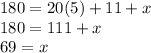Answer:
x = 5; m<1 = 69
Explanation:
We know that the two angles with degrees 20x+11 and 25x-14 are vertical angles and are thus congruent or equal. Therefore, we can set the two angles equal to each other to find x:
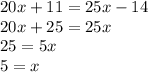
If we plug in x into any of the two angles, we find the measure of both angles is 111.
The most probable relationship between angle 1 and the other two angles is supplementary, which means that the measure of angle 1 and any of the two angles beside it is 180.
To find m<1, represented as x, we can use the equation: