Question

Help please
Help please
Help please
Help please

Answer 1

Given:

The four table of values.

To find:

The table whose linear function has slope 2.

Solution:

Slope formula:

`m=(y_2-y_1)/(x_2-x_1)`

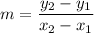
Consider any two points from each table and find the slope for each table.

For table 1, the two points are (2,1) and (6,-1). So, the slope of the linear function is:

`m=(-1-1)/(6-2)`

`m=(-2)/(4)`

`m=(-1)/(2)`

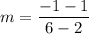
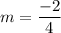
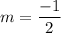
For table 2, the two points are (0,8) and (2,4). So, the slope of the linear function is:

`m=(4-8)/(2-0)`

`m=(-4)/(2)`

`m=2`

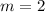
For table 3, the two points are (-4,4) and (-2,5). So, the slope of the linear function is:

`m=(5-4)/(-2-(-4))`

`m=(1)/(-2+4)`

`m=(1)/(2)`

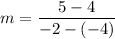
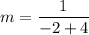
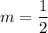
For table 4, the two points are (-2,0) and (0,4). So, the slope of the linear function is:

`m=(4-0)/(0-(-2))`

`m=(4)/(2)`

`m=2`

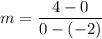
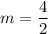
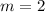
Table 4 is the only table that represents a linear function whose slope is 2.

Therefore, the correct option is 4, Table 4.