Answer:
A. Circle.
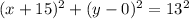
B. Parabola.
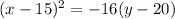
C. Maximum height of Tunnel A = 13 ft.
Maximum height of Tunnel B = 20 ft.
The truck can only pass through Tunnel B without damage.
Explanation:

Circle: A and C are non-zero and equal, and have the same sign.
Ellipse: A and C are non-zero and unequal, and have the same sign.
Parabola: A or C is zero.
Hyperbola: A and C are non-zero and have different signs.
Part A
Tunnel A
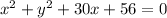
As the coefficients of x² and y² are non-zero, equal and have the same sign, the conic section is a circle.

Rewrite the given equation for Tunnel A in the standard form of the equation of a circle:
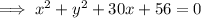
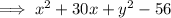

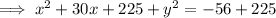

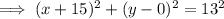
Therefore, the center of the circle is (-15, 0) and the radius is 13.
Part B
Tunnel B
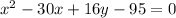
There is no term in y² so the coefficient of y² is zero. Therefore, the conic section is a parabola.

Rewrite the given equation for Tunnel B in the standard form a parabola:
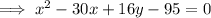
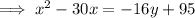

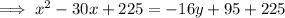
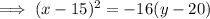
Therefore, the vertex is (15, 20).
Part C
Maximum height of Tunnel A
The maximum point of a circle is the sum of the y-value of its center and its radius:
Maximum height of Tunnel B
The maximum point of a downwards opening parabola is the y-value of its vertex:
As the truck is 13.5 feet high, it cannot pass through Tunnel A since the maximum height of Tunnel A is 13 feet.
The maximum height of Tunnel B is certainly adequate for the truck to pass through. However, to determine if the truck can pass through Tunnel B safely, we also need to find the width of the tunnel when its height is 13.5 feet. To do this, find the x-values of the parabola when y = 13.5. If the difference in x-values is 8 or more, then the truck can pass through safely.
Substitute y = 13.5 into the equation for Tunnel B and solve for x:
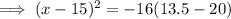
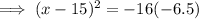
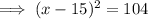
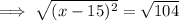

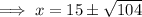
Now find the difference between the two found values of x:
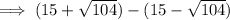

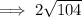

Therefore, as the width of Tunnel B is 20.4 ft when its height is 13.5 ft, the 8 ft wide truck can easily pass through without damage since 20.4 ft is greater than the width of the truck.