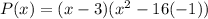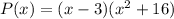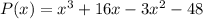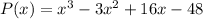Answer:
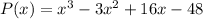
Explanation:
Given information:
- Polynomial function with real coefficients.
- Zeros: 3 and 4i
- Lead coefficient of 1.
For any complex number
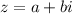 , the complex conjugate of the number is defined as
, the complex conjugate of the number is defined as
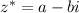 .
.
If f(z) is a polynomial with real coefficients, and z₁ is a root of f(z)=0, then its complex conjugate z₁* is also a root of f(z)=0.
Therefore, if P(x) is a polynomial with real coefficients, and 4i is a root of f(x)=0, then its complex conjugate -4i is also a root of P(x)=0.
Therefore, the polynomial in factored form is:

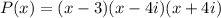
Expand the polynomial: