Question

Suppose that $36,000 is deposited in an account and the balance increases to $39,786.15 after 2.5 years. how long will it take for the account to grow to $51,806.67? Assume continous compounding

It will take about _ years for $36,000 to grow to $51,806.67

Answer 1

9 years

Explanation:

Continuous Compounding Formula

`\large \text{$ \sf A=Pe^(rt) $}`

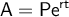
where:

• A = Final amount.
• P = Principal amount.
• e = Euler's number (constant).
• r = Annual interest rate (in decimal form).
• t = Time (in years).

Given values:

• P = $36,000
• A = $39,786.15
• t = 2.5 years

Substitute the given values into the formula and solve for r to find the annual interest rate:

`\implies \sf 39786.15=36000 \cdot e^(2.5r)`

`\implies \sf (39786.15)/(36000)= e^(2.5r)`

`\implies \sf \ln \left((39786.15)/(36000)\right)=\ln e^(2.5r)`

`\implies \sf \ln \left((39786.15)/(36000)\right)=2.5r`

`\implies \sf (1)/(2.5)\ln \left((39786.15)/(36000)\right)=r`

`\implies \sf r=(2)/(5)\ln \left((39786.15)/(36000)\right)`

`\implies \sf r=0.03999996933...`

`\implies \sf r=0.04\;(2\;d.p.)`

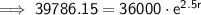
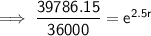
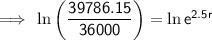
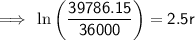
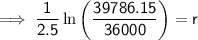
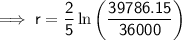
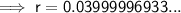
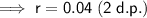
Therefore, the annual interest rate is 0.04 = 4%.

To calculate how many years it will take for $36,000 to grow to $51,806.67, substitute the values into the formula and solve for t:

`\implies \sf 51806.67=36000 \cdot e^(0.04t)`

`\implies \sf (51806.67)/(36000)= e^(0.04t)`

`\implies \sf \ln\left((51806.67)/(36000)\right)= \ln e^(0.04t)`

`\implies \sf \ln\left((51806.67)/(36000)\right)= 0.04t`

`\implies \sf (\ln\left((51806.67)/(36000)\right))/(0.04)=t`

`\implies \sf t=9.10 \; (2\;d.p.)`

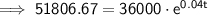
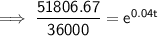
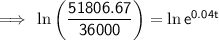
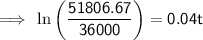
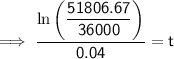
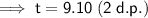
Therefore, it will take about 9 years for $36,000 to grow to $51,806,67.

Answer 2

• About 9 years

===================================

Continuous compounding equation:

• 
  `P(t) = P_0e^(rt)`

Given

• 
  `P_0=36000`
• 
  `P(t)=39.876.15`
• 
  `t=2.5`

Find the value of r

• 
  `39786.15= 36000*e^(r*2.5)`
• 
  `e^(2.5r)=39786.15/36000`
• 
  `ln \ e^(2.5r)= ln \ 1.10517`
• 
  `2.5r=0.099`
• 
  `r=0.099/2.5`
• 
  `r=0.04`

Now find the time t for the balance to grow to $51806.67:

• 
  `51806.67= 36000*e^(0.04t)`
• 
  `51806.67/36000=e^(0.04t)`
• 
  `e^(0.04t)= 1.439`
• 
  `ln \ e^(0.04t)= ln \ 1.439`
• 
  `0.04t=0.3639`
• 
  `t=0.3639/0.04`
• 
  `t=9.0975`

The time required is about 9 years.