Question

A family is relocating from St. Louis, Missouri, to California. Due to an increasing inventory of houses in St. Louis, it is taking longer than before to sell a house. The wife is concerned and wants to know when it is optimal to put their house on the market. Her realtor friend informs them that the last 22 houses that sold in their neighborhood took an average time of 190 days to sell. The realtor also tell them that based on her prior experience, the population standard deviation is 72 days. Assume the number of days to sell a house has a normal distribution Find the lower bound of the 95% confidence interval for the mean sale time for all homes in the neighborhood.

Answer 1

The lower bound of the 95% confidence interval for the mean sale time for all homes in the neighborhood is of 160 days.

Explanation:

We have that to find our
`\alpha` level, that is the subtraction of 1 by the confidence interval divided by 2. So:

`\alpha = (1 - 0.95)/(2) = 0.025`

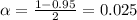
Now, we have to find z in the Ztable as such z has a pvalue of
`1 - \alpha`.

That is z with a pvalue of
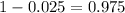`1 - 0.025 = 0.975`, so Z = 1.96.

Now, find the margin of error M as such

`M = z(\sigma)/(√(n))`

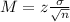
In which
`\sigma` is the standard deviation of the population and n is the size of the sample.

`M = 1.96(72)/(√(22)) = 30`

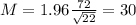
The lower end of the interval is the sample mean subtracted by M. So it is 190 - 30 = 160 days

The lower bound of the 95% confidence interval for the mean sale time for all homes in the neighborhood is of 160 days.