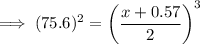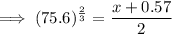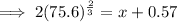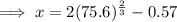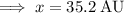Question

I am not sure how to approach this question.

Answer 1

35.2 AU

Step-by-step explanation:

Kepler's Third Law states that the square of the orbital period of a planet is directly proportional to the cube of the semi-major axis of its orbit.

`\boxed{T^2 \propto a^3 \implies T^2=ka^3}`

Kepler's Third Law

`T^2=(4 \pi^2)/(GM)a^3`

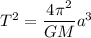
where a is the semi-major axis of the ellipse.

If expressed in the following units:

• T = Earth years.
• a = Astronomical units AU (a=1 AU for Earth).
• M = Solar masses.

then:

`\implies(4 \pi^2)/(GM)=1`

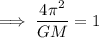
Therefore Kepler's Third Law can be expressed as:

`\boxed{T^2=a^3}`

Given:

• T = 75.6 years

From inspection of the given diagram:

`\implies 2a=x+0.57`

`\implies a=(x+0.57)/(2)`

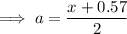
Substitute these values into the equation and solve for x:

`\implies (75.6)^2=\left((x+0.57)/(2)\right)^3`

`\implies (75.6)^(2)/(3)=(x+0.57)/(2)`

`\implies 2(75.6)^(2)/(3)=x+0.57`

`\implies x=2(75.6)^(2)/(3)-0.57`

`\implies x=35.1883819...`

`\implies x=35.2\; \text{AU}`