Question

In a given arithmetic progression, the first term is 6, and the 87-th term is 178. Find the common

difference of this arithmetic progression and give the value of the first five terms.

Answer 1

d = 2 and terms are 6, 8, 10 12 and 14

Explanation:

we know

`a_(n) = a_(1) + (n-1)d`

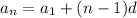
here a(n) = nth term

a1 = 1st term

n = no. of term

d = common difference

putting the values we get,

178 = 6 + (87-1)d

⇒ 178 = 6 + 86d

⇒86d = 172

⇒ d = 2

So required common difference is 2.

Now the first term is 2

so second term is a2 = 6 + ((2-1)×2) = 8

third term = 6 + ((3-1)×2) = 10

fourth term = 6 + ((4-1)×2) = 12

fifth term = 6 + ((5-1)×2) = 14