Question

Julie buys her jacket after the four reductions 25% what percentage of the original price 37 $ dose she save

Answer 1

let's say "x" is the original price, now let's reduce it by 25%

`\begin{array}c \cline{1-1} \textit{\textit{\LARGE a}\% of \textit{\LARGE b}}\\ \cline{1-1} \\ \left( \cfrac{\textit{\LARGE a}}{100} \right)\cdot \textit{\LARGE b} \\\\ \cline{1-1} \end{array}~\hspace{5em}\stackrel{\textit{25\% of x}}{\left( \cfrac{25}{100} \right)x}\implies \cfrac{1}{4}x\hspace{5em}x-\stackrel{25\%}{\cfrac{1}{4}x}\implies \stackrel{new~price}{\cfrac{3}{4}x}`

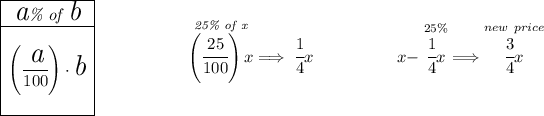
so the new price after applying a 25% or namely 1/4 of "x" is just 3/4 of "x".

if we apply the same reduction again, we'll be left with only 3/4 of ( (3/4)x ) and so on, check the picture below which shows the value once we apply the 1/4 four times, we end up with the new price being to 3/4 of whatever it was before, so

`\cfrac{3}{4}\cdot \cfrac{3}{4}\cdot \cfrac{3}{4}\cdot \cfrac{3}{4}x\implies \cfrac{81}{256}x\hspace{5em}\cfrac{81}{256}\cdot 37\implies \cfrac{2997}{256}`

now, since we know the 100% or original price was 37, what's the new reduced price of 2997/256 off of it in percentage?

`\begin{array}{ccll} amount\%\\ \cline{1-2} 37&100\\[1em] (2997)/(256)&p \end{array}\implies \cfrac{37}{~~ (2997 )/(256 ) ~~}=\cfrac{100}{p}\implies \cfrac{(37)(256)}{2997}=\cfrac{100}{p} \\\\\\ (37)(256)p=299700\implies p=\cfrac{299700}{(37)(256)}\implies p\approx \stackrel{\%}{31.64}~\hfill \underset{savings}{\stackrel{100\%~~ - ~~31.64\%}{\approx\text{\LARGE 68.36\%}}}`