Question

NO LINKS!! Please help me with these graphs Part 3​

Answer 1

`\textsf{a.} \quad x = 1 - √(7),\quad x = 1 + √(7)`

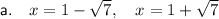
b. y = -6

c. Minimum point at (1, -7).

d. Domain: (-∞, ∞)

e. Range: [-7, ∞)

f. See attachment.

Explanation:

Given function:

`\text{f}(x)=x^2-2x-6`

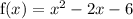
As per the question, use a graphing calculator to graph the given function.

Part a

The x-intercept(s) are the points at which the curve crosses the x-axis.

• x-intercepts:
  `x = 1 - √(7),\quad x = 1 + √(7)`
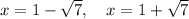
  These are x = -1.6 and x = 3.6 to one decimal place.

Part b

The y-intercept is the point at which the curve crosses the y-axis.

• y-intercept: y = -6

Part c

From inspection of the graph:

• Minimum point at (1, -7).

Part d

The domain of a function is the set of all possible input values (x-values).

The domain of the given function is unrestricted.

• Domain: (-∞, ∞)

Part e

The range of a function is the set of all possible output values (y-values).

As the function has a minimum point at y = -7, the range is restricted.

• Range: [-7, ∞)

Part f

Graph label the axes using a scale of 1 (see attachment).

• Plot the minimum point (1, -7).
  The axis of symmetry is the x-value of the vertex: x = 1.

• Plot the x-intercepts: (-1.6, 0) and (3.6, 0)

• Plot the y-intercept: (0, -6)
• Using the axis of symmetry to ensure the curve is symmetrical, draw a curve through the plotted points.