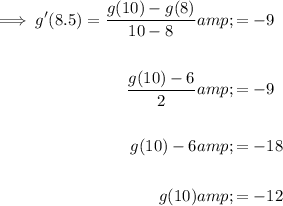Question

The function g(x) is continuous on the closed interval [8, 10] and differentiable on the open interval

(8, 10). The value x = 8.5 satisfies the conditions of the Mean Value Theorem on the interval [8, 10].
What is g(10) given g'(8.5) = -9 and g(8) = 6?

Answer 1

g(10) = -12

Explanation:

Mean Value Theorem

If f is continuous on [a, b] and differentiable on (a, b), then there is a number c such that a < c < b and:

`f'(c)=(f(b)-f(a))/(b-a)`

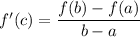
We are told that the function g(x) is continuous on the closed interval [8, 10] and differentiable on the open interval (8, 10).

Therefore:

• a = 8
• b = 10

Given:

• g'(8.5) = -9
• g(8) = 6

As 8 < 8.5 < 10 then c = 8.5:

`\begin{aligned} \implies g'(8.5)=(g(10)-g(8))/(10-8)&=-9\\\\(g(10)-6)/(2)&=-9\\\\g(10)-6&=-18\\\\g(10)&=-12\end{aligned}`