Question

The angles of a triangle are in the ratio 1:1:2. If the largestside of the triangle is 4, then the shortest side of the triangle is

Answer 1

Triangle of sides ratio 1 : 1 : 2 is an isosceles triangle, with a diagram given above to illustrate it.

With the ratio of the sides given,

Largest side of the triangle is 4 units, let the length of the shortest side be x

Using scale ratio to determine the shortest side,

`\begin{gathered} \text{Scale ratio of the sides =}\frac{shortest\text{ side}}{largest\text{ side}}=(1)/(2) \\ \text{Actual length ratio = }\frac{shorstest\text{ side}}{largest\text{ side}}=(x)/(4) \\ \text{Equating both ratios} \\ (x)/(4)=(1)/(2) \\ \text{Crossmultiply} \\ 2* x=4*1 \\ 2x=4 \\ \text{Divide both sides by 2} \\ (2x)/(2)=(4)/(2) \\ x=2 \end{gathered}`

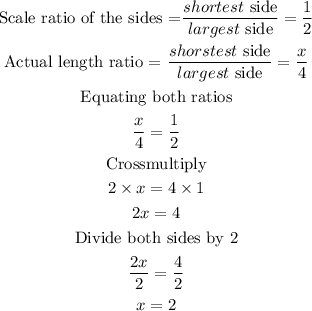
Hence, the length of the shortest side is 2 units.