We will determine the number of ways a group of 4 can be chosen as follows:
*First: We will have to take into account the follwing:
When we start choosing we will have 15 total people to choose from, when we choose the second one we will have 14 people to choose from and so on, thus:
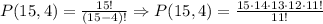
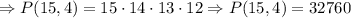
So, there are 32 760 different ways a group of 4 can be choosen at random.