Question

A quadratic function f is given by f(x)=ax2+bx+c where a is not 0. Select all the statements that must be true about the graph of f.

Answer 1

Given the quadratic equation y = ax² + bx + c, let's evaluate each sentence.

(a) The y-intercept is the point (0, c).

The y-intercept is a point (0, y). Substituting x by 0, we have:

`\begin{gathered} y=a*0+b*0+c \\ y=c \end{gathered}`

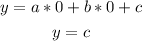
So, the y-intercept is the point (0, c).

The statement is TRUE

(b) The graph as a x-intercept (c, 0).

The x-intercept is the point (x, 0). Substituting y by 0, we have:

`\begin{gathered} y=ax^2+bx+c \\ 0=ax^2+bx+c \end{gathered}`

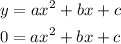
So, the statement is FALSE.

(c) When a < , the function opens downtown.

When a < 0, the graph is:

So, the statement is TRUE.

(d) The graph has two x-intercepts.

The graph can have 0, 1 or 2 y-intercepts.

So, the statement is FALSE.

(e) If b = 0, then the vertex is on the x-axis.

When b = 0, then the vertex is on the x-axis. TRUE.