Question

4. Law of Acceleration A 1500 kg car is forced to stop suddenly. If it was originally moving at 16 m/s and stops ina time of 4.7...What is the car's acceleration?How much net force is required?

Answer 1

In order to determine the acceleration of the car use the following formula:

`v=v_o-at_{}`

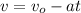
where,

vo: initial velocity = 16 m/s

v: final velocity = 0 m/s

t: time = 4.7 s

a: acceleration = ?

Solve the equation above for a and replace the values of the other parameters:

`a=(v_o-v)/(t)=((16m)/(s)-(0m)/(s))/(4.7s)=(3.40m)/(s^2)`

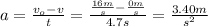
Hence, the acceleration of the car is 3.40 m/s^2, or if you consider that the car is deccelerating, it is -3.40 m/s^2.

To calculate the net required force, use the Newton second law:

`F=ma`

where,

m: mass of the car = 1500 kg

F: force = ?

Replace the previous values of the parameters into the expression for F:

`F=(1500kg)((3.40m)/(s^2))=5100N`

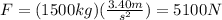
Hence, the required force is 5100N