Question

The forklift exerts a 300.0 N force on the box and moves it 15.0 m forward to the stack.How much work does the forklift do against the force of gravity?

Answer 1

Given data:

A force exerts by forklift on the box,

`F=300.0\text{ N}`

The displacement of box,

`d=15\text{ m}`

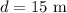
The formula of work done is as follows:

`W=Fd\cos \theta`
`\text{Here, }\theta\text{ is an angle betw}een\text{ force and displacement}`

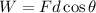
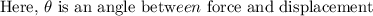
In the given case,

Box is moving in forward direction (horizontal direction) and gravitation force exerts on a box is in the downwards direction.

Therefore, an angle between gravitataional force and displacement of box is,

`\theta=90^o`

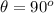
Substitute known values in above equation;

`\begin{gathered} W=Fd\cos 90^o \\ W=0\text{ J} \end{gathered}`

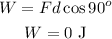
Therefore, the work done by forklift against the gravity is 0 J