We are asked to determine the area of the sector of a circle with a redius of 9 meters and an angle of 90 degrees. To do that we will use the following formula:
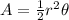
Where:
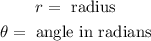
We have that 90 degrees in radians are equivalent to:

Substituting the values in the formula:
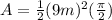
Solving the operations:

Therefore, the area is approximately 63.62 square meters.