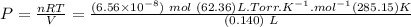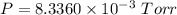Question

A 140 mL flask contains 2.1 micrograms of O2 at 12°C.What is the pressure?Answer in units of Torr.

Answer 1

`P=8.3360*10^(-3)\text{ }Torr`

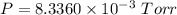
Procedure

The gas can be considered ideal given the temperature conditions. Therefore we can use the ideal gas formula

`PV=nRT`

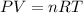
Before substituting the values we need the moles of oxygen

`2.1*10^(-6)g\text{ }O_2\frac{1mole\text{ }O_2}{31.999g\text{ }O_2}=6.56*10^(-8)\text{ }mole\text{ }O_2`

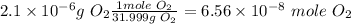
Then we need the gas constant that contains Torr as pressure units

R=62.363577 L.Torr.°K⁻¹.mol⁻¹

We need to convert the volume and temperature into the constant units

T= 12°C+273.15=285.15°K

V=140mL=0.140L

Now we can proceed to substitute the values in the ideal gas equation

`P=(nRT)/(V)=\frac{(6.56*10^(-8))\text{ }mol\text{ }(62.36)L.Torr.\degree K^(-1).mol^(-1)(285.15)\degree K}{(0.140)\text{ }L}`
`P=8.3360*10^(-3)\text{ }Torr`