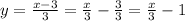Question

What function is graphed below?y = x + 3y = x/3 - 1y = 3 xy = x - 3

Answer 1

Given:

There is a graph given

Required:

We need to find that which option is the function of graph

Step-by-step explanation:

Here by the graph we can say that

`\begin{gathered} (x_1,y_1)=(3,0) \\ (x_2,y_2)=(6,1) \end{gathered}`

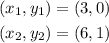
First of all we need to find the slope m of graph

`m=((y_2-y_1))/((x_2-x_1))=(1-0)/(6-3)=(1)/(3)`

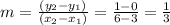
now the equation of line is

`\begin{gathered} m=(y-0)/(x-3) \\ \\ (1)/(3)=(y)/(x-3) \\ \\ (x-3)/(3)=y \end{gathered}`

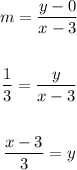
Final answer:

`y=(x-3)/(3)=(x)/(3)-(3)/(3)=(x)/(3)-1`
`y=(x)/(3)-1`