Question

what is the equation for the graph below? A y = − (x − 3)2 + 1B y = − (x + 3)2 + 1C y = (x − 3)2 − 1D y = (x + 3)2 − 1

Answer 1

Notice that the graph passes through the two points (0,2) and (-2,8)

The equation of the straight line graph will be of the form

`y=mx+c`

Where m is the slope and c is the y - intercept

We will first the slope first

`m=(y_2-y_1)/(x_2-x_1)`
`\begin{gathered} x_1=0 \\ y_1=2_{} \\ x_2=-2 \\ y_2=8 \end{gathered}`
`\begin{gathered} m=(y_2-y_1)/(x_2-x_1) \\ m=(8-2)/(-2-0) \\ m=(6)/(-2) \\ m=-3 \end{gathered}`

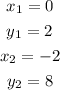
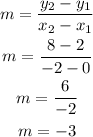
Thus,

`y=-3x+c`

Recall that c is the y - intercept and the y - intercept is at 2

Thus,

`c=2`
`y=-3x+2`

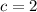
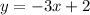
We will now consider the options to see which gives the above equation

Option A

`\begin{gathered} y+1=-3(x-1) \\ y+1=-3x+3 \\ y=-3x+3-1 \\ y=-3x+2 \end{gathered}`

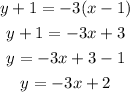
Therefore, Option A is Correct