Question

As seen in the accompanying diagram, a person can travel from New York City to Bufalo by going north 170 miles to Albany and then west 280 miles to Buffalo Buffalo If an engineer wants to design way to connect New York City directly to Buffalo what angle, would she need to build the highway? Find the angle to the nearest degree. To the serie how many miles would be saved by traveling directly from New York City to Buffalo rather than by traveling first to Albany and then to Buffalo

Answer 1

Anglex is 58.74 degre.

Miles saved by travelling in highways is 122.43 miles.

Given:

Distance between New York to Alban is 170 miles.

Distance between Alban to Baffalo is 280 miles.

The objective is to find the angle of highway to be built directly from New York to Buffalo. and also the difference in mile between the two routes.

The given image can be represented as,

In the obtained triangle right angled at B, as angle is required at A, then AC represents the hypotenuse side, AB represents the adjacent side and BC represents the opposite side.

Now,the angle can be calculated by the opposite side and adjacent side using tan theta.

`\begin{gathered} \tan \theta=(opposite)/(adjacent) \\ \tan x=(280)/(170) \\ x=\tan ^(-1)((280)/(170)) \\ x=59^0 \end{gathered}`

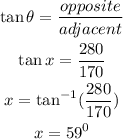
Now, the difference between the two routes can be calculated using Pythagorean theorem of the right triangle.

`\begin{gathered} \text{Hypotenuse}^2=Opposite^2+Adjacent^2 \\ AC^2=BC^2+AC^2 \\ AC^2=280^2+170^2 \\ AC^2=78400+28900 \\ AC^2=107300 \\ AC=\sqrt[]{107300} \\ AC=327.57\text{ miles} \end{gathered}`

Thus the distance of highways directly from New york to Buffalo is 327.57 miles.

Now, consider D as the difference miles that would be saved by traveling directly from New York City to Buffalo

Then, the miles saced by travelling in this highway is,

`\begin{gathered} D=(AB+BC)-AC \\ D=(280+170)-327.57 \\ D=450-327.57 \\ D=122.43\text{ miles} \end{gathered}`

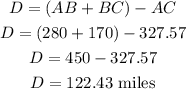
Hence, the angle required to build the highway is 59 degree. And by traveling in this highway directly from New york city to Buffalo will save 122.43 miles.