EXPLANATION
First, we need to apply the compounding interest equation as given below:

Where A=balance, P=principal, r=interest rate, n=number of times interest rate is compounded (in this case the interest rate is compounded annually, so n=1)
First, we need to isolate the interest rate from each bank.
Isolating r from the Compounding interst equation:
(Dividing both sides by P):

Applying the nt root to both sides:
![\sqrt[nt]{(A)/(P)}=(1+(r)/(n))](https://img.qammunity.org/2023/formulas/mathematics/college/dhdbs8r6rh9joue75j6amvg95n37dqeyun.png)
Removing the parentheses:
![\sqrt[nt]{(A)/(P)}=1+(r)/(n)](https://img.qammunity.org/2023/formulas/mathematics/college/ibhfwrjhrrn4pdho0cnzcz66xqtpb83fuw.png)
Subtracting -1 to both sides:
![\sqrt[nt]{(A)/(P)}-1=(r)/(n)](https://img.qammunity.org/2023/formulas/mathematics/college/6nvyx2jpy5c1sbv8v81kajgnthkev7lg9q.png)
Multiplying both sides by n. As n=1 we can desestimate this step.Additionally, as n=1 --> nt=1*t = t
![\sqrt[t]{(A)/(P)}-1=r](https://img.qammunity.org/2023/formulas/mathematics/college/hy3ofoqfgqhw50sjk1oknaxlhjake4gtms.png)
Switching sides:
![r=\sqrt[t]{(A)/(P)}-1](https://img.qammunity.org/2023/formulas/mathematics/college/ep2mvl1zr22rskz6p5l7dbsd3kpytszgrs.png)
Now we can compute the interest rate for each bank as follows:
![r_{Super\text{ Save}}=\sqrt[6]{(1173.34)/(1000)}-1](https://img.qammunity.org/2023/formulas/mathematics/college/54ugu2sn9ls89u0byaznpladlgyk8x798c.png)
![r_{\text{Super Save}}=\sqrt[6]{1.17334}-1](https://img.qammunity.org/2023/formulas/mathematics/college/swlzhzbhpt0sfegod0tmwh33nqz13z4r3f.png)
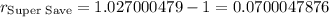
As r is represented in decimal form, the r_Super Save= 2.70%
Applying the same reasoning to Star Financial:
![r_{\text{Star Financial}}=\sqrt[3]{(2684.35)/(2500)}-1](https://img.qammunity.org/2023/formulas/mathematics/college/pro19x3iom00ifm39u9dfwyfiujw9kjprf.png)
![r_{\text{Star Financial}}=\sqrt[3]{1.07374}-1](https://img.qammunity.org/2023/formulas/mathematics/college/rgbjzm6dfihvcjxvm89awbgpbo5tduf8be.png)
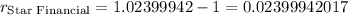
As r is represented in decimal form, the r_Star Financial= 2.39%
Applying the same reasoning to Better Bank:
![r_{\text{Better Bank}}=\sqrt[5]{(4525.63)/(4000)}-1](https://img.qammunity.org/2023/formulas/mathematics/college/6g43m7skzpkn4h8xni5adofy7rsfnst0ep.png)
![r_{\text{Better Bank}}=\sqrt[5]{1.1314075}-1](https://img.qammunity.org/2023/formulas/mathematics/college/14vdxnsnlo9tpj2kc79ofi9i5odn3a0ps0.png)
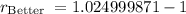
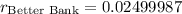
As r is represented in decimal form, the r_Better Bank= 2.49%
Now, that we have the interest rate values, we can build a table for each year corresponding to each Bank:
In order to draw the graph and using the table, we need to compute the balance for each year applying the above equation:
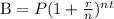
Note: (Commas and periods are reversed, Spreadsheet Configuration issue)