Question

3. Scores on the SAT form a normal distribution with a mean of 500 and StandardDeviation of 100.a) What is the minimum score necessary to be in the top 10% of the SATdistribution?b) Find the range of values that defines the middle 80% of the distribution ofSAT scores (372 and 628). (The z scores)

Answer 1

Given

Mean is 500 and Standard Deviation is 100.

Part A

Using Z- Score

The minimum score necessary to be in the top 10% of the SAT distribution means 90% and above

`\begin{gathered} Z=(x-\mu)/(\sigma) \\ \\ x=0.9 \\ \mu=500 \\ \sigma=100 \\ 1.282=(x-500)/(100) \\ \\ x=628.2 \end{gathered}`

Thus, the minimum score is 628. To the nearest whole number.

Part B

We want to determine the range of values that correspond to the probability;

`Pr(-ZThe z scores corresponding to this is; [tex]z=+1.282\text{ }&-1.282`

Thus;

`\begin{gathered} 1.282=(x-500)/(100)\text{ and }-1.282=(x-500)/(100) \\ 628.2=x\text{ }and\text{ }371.8 \end{gathered}`

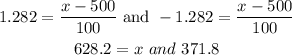
Thus, the range of scores are 372 and 628