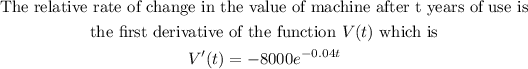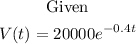
a) What is the depreciation rate after 5 years of use?
First, get the derivative of the function V(t), and then substitute t = 5.


The result is negative since the value is depreciating. We can therefore, conclude that the depreciation rate after 5 years of use is $1082.68.
b) What is the relative rate of change in the value of the machine after t years of use.