Question

This is a Math practice worksheet called PERMUTATIONS,COMBINATIONS, AND FUNDAMENTAL COUNTING PRINCIPLE PRACTICE. The question is: How many arrangements can be formed using the letters in the word AUSPICIOUS?

Answer 1

453,600 arrangements

Step-by-step explanation

When one is asked to find the number of arrangements of the letters of a given word (with n letters), the number of arrangements is calculated as

`\text{Number of arrangements =}(n!)/(k_1!k_2!k_3\ldots.)`

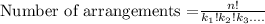
where k₁, k₂, k₃ are the number of times that letters that occur multiple times appear.

For this question and for the word AUSPICIOUS,

The number of letters in the word is 10 letters.

And the letters, U, S and I all occur 2 times each.

n = 10

k₁ = 2, k₂ = 2, k₃ = 2

Number of arrangements

= 10! ÷ [(2!)(2!)(2!)]

= 3,628,800 ÷ 8

= 453,600 arrangements

Hope this Helps!!!