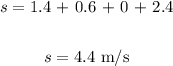Question

Someone please help me into what was the average speed for the entire trip thank you

Answer 1

From the position-time graph, we have the following:

Distance covered Time

0 to 28 m 0 to 20 seconds

28m to 52 m 20 to 60 seconds

At 52 m 60 to 80 seconds

52 to -20m 80 to 110 seconds

Let's find the average speed for the entire trip.

To find the average speed, apply the formula:

`avg\text{ spe}ed=\frac{total\text{ distance}}{total\text{ time}}`

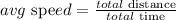
Distance at line 1: 28 - 0 = 28m

Distance at line 2: 52 - 28 = 24 m

Distance at line 3: 0 m

Distance at line 4: -20m - 52 = -72 m

Thus, we have the equation:

`s=(d_1)/(t_1)+(d_2)/(t_2)+(d_3)/(t_3)+(d_4)/(t_4)`

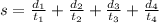
Where:

d1 = 28 m

d2 = 24 m

d3 = 0 m

d4 = 72 m

t = 110 s

Now substitue values into the equation:

`\begin{gathered} s=(28)/(20)+(24)/(40)+(0)/(20)+(72)/(30) \\ \\ s=4.4\text{ m/s} \end{gathered}`

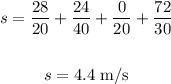
Therefore, the average speed for the trip is 4.4 m/s.

ANSWER:

Equation:

`s=(d_1)/(t_1)+(d_2)/(t_2)+(d_3)/(t_3)+(d_4)/(t_4)`

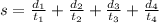
Substitution:

`s=(28)/(20)+(24)/(40)+(0)/(20)+(72)/(30)`

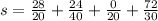
Calculation:

`\begin{gathered} s=1.4\text{ + }0.6\text{ + 0 + }2.4 \\ \\ s=4.4\text{ m/s} \end{gathered}`