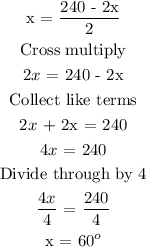Step 1: Theorem
The measure of the angle between two tangent that intercept outside a circle is half the positive difference of the measure of the intercepted arcs.
Step 2: Redraw the figure.
Step 3:
We know that 2x + 240 = 360.
Collect like terms
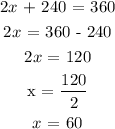
The value of x can also be calculated using the formula below from the theorem.
The measure of the angle between two tangent that intercept outside a circle is half the positive difference of the measure of the intercepted arcs