Question

Malcolm is making 12 pounds of fruit salad with pineapple and strawberries. Pineapples cost $7.50 per pound and strawberries cost $10.50 per pound. How many pounds of pineapple and how many pounds of strawberries should Malcolm use for the mixture to cost $9 per pound to make?

Answer 1

6 pounds of strawberry

6 pounds of pineapples

Step-by-step explanation:

Let,

s = number of pounds of strawberry

p = number of pounds of pineapples

Now we are told that Malcolm is making 12 pounds of fruit salad with pineapple and strawberries. This means

`s+p=12`

Furthermore, we are also told that Pineapples cost $7.50 per pound and strawberries cost $10.50 per pound. This means that the cost of the mixture will be

`7.50p+10.50s`

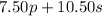
Therefore, the cost per pound of the mixture is

`(7.50p+10.50s)/(12)`

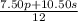
which we are told is $9 per pound. Therefore,

`(7.50p+10.50s)/(12)=9`

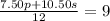
We can multiply both sides of the above equation by 12 and get:

`7.50p+10.50s=9*12`
`7.50p+10.50s=108`

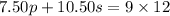
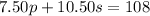
Hence, we have two equations and two unknowns:

`\begin{gathered} p+s=12 \\ 7.50p+10.50s=108 \end{gathered}`

To solve the above system for s and p, we first solve for p in the first equation.

Subtracting s from both sides of the first equation gives

`\begin{gathered} p+s=12 \\ \Rightarrow p=12-s \end{gathered}`

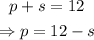
Substituting this value of p in the second equation gives

`7.50(12-s)+10.50s=108`

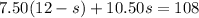
which we expand to get

`90-7.50s+10.50s=108`
`90+3s=108`

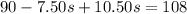
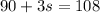
Subtracting 90 from both sides gives

`3s=108-90`
`3s=18`

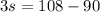
Finally, dividing both sides by 3 gives

`s=18/3`
`\boxed{s=6.}`

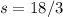
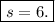
WIth the value of s in hand, we now find p.

`p+s=12`

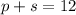
Putting s = 6 into the above equation gives

`p+6=12`

subtracting 6 from both sides gives

`\boxed{p=6.}`

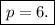
Hence, s = 6 and p = 6. This means, 6 pounds of strawberry and 6