The area of any sector of a circle is
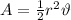
Where r is the radius of the circle and theta is the central angle of the sector in the radian measure
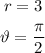
Substitute them in the rule above
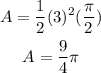
The area of the top wedge is 9/4 pi square inches
You can use pi = 3.14
A = 7.065 square inches