This is a question of matched samples with continuous outcomes where we compare the means of two results gotten by people in a survey before and after a test.
We are asked to find:
1. Test statistic
2. P-value
3. Compare the p-value with an alpha level
4. Decision to make given the p-value.
To get started on solving this question, there is one formula we need to know. This formula is:
![\begin{gathered} Z_{\text{stat}}=\frac{\bar{X}-\mu_d}{\frac{s_d}{\sqrt[]{n}}} \\ \text{where,} \\ \bar{X}_d=\text{sample difference} \\ \mu_d=\text{population difference} \\ s_d=\text{sample difference standard deviation} \\ n=\text{ number of samples in the survey} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/tda937evik5jr43nol4hjyn3qirmka7r3q.png)
(Note, we are using Z-statistic because the number of people in the survey is more than 30. If not, we would have used T-statistic)
With this formula, we can begin solving.
1. Test Statistic:
![\begin{gathered} \bar{X}_d=-2.1 \\ \mu_d=0 \\ s_d=29.3 \\ n=335 \\ \\ \therefore Z_{\text{stat}}=\frac{-2.1-0}{\frac{29.3}{\sqrt[]{335}}}=-1.312 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/p7am892ps67kjd3nreeq289e065t7j25uu.png)
Thus, the value of the test statistic is -1.312 (To 3 decimal places)
2. P-value:
We can check the p-value corresponding to the test statistic using Z-distribution tables.
Checking the value -1.312 on the Z-distribution table, we have:
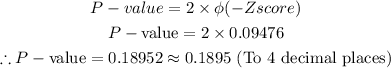
(We multiply by two because this is a two-tailed test)
3. P-value Comparison:
The p-value of 0.1895 is much greater than the alpha level of 0.001